Twin Primes

The Prime Pages keeps a list of the 5000 largest known primes, plus a few each of certain selected archivable forms and classes. These forms are defined in this collection's home page.

This page is about one of those forms.

Definitions and Notes

Twin primes are pairs of primes which differ by two. The first twin primes are {3,5}, {5,7}, {11,13} and {17,19}. It has been conjectured (but never proven) that there are infinitely many twin primes. If the probability of a random integer n and the integer n+2 being prime were statistically independent events, then it would follow from the prime number theorem that there are about n/(log n)² twin primes less than or equal to n. These probabilities are not independent, so Hardy and Littlewood conjectured that the correct estimate should be the following.

Here the infinite product is the twin prime constant (estimated by Wrench and others to be approximately 0.6601618158...), and we introduce an integral to improve the quality of the estimate. This estimate works quite well! For example: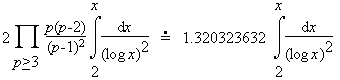

The number of twin primes
less than N

N	actual	estimate
106	8169	8248
108	440312	440368
1010	27412679	27411417

There is a longer table by Kutnib and Richstein available online.

In 1919 Brun showed that the sum of the reciprocals of the twin primes converges to a sum now called Brun's Constant. (Recall that the sum of the reciprocals of all primes diverges.) By calculating the twin primes up to 10¹⁴ (and discovering the infamous pentium bug along the way), Thomas Nicely heuristically estimates Brun's constant to be 1.902160578.

As an exercise you might want to prove the following version of Wilson's theorem.

Theorem: (Clement 1949)

The integers n, n+2, form a pair of twin primes if and only if

4[(n-1)!+1] ≡ -n (mod n(n+2)).

Nice--too bad it is of virtually no practical value!

Record Primes of this Type

rank prime digits who when comment 1 2996863034895 · 21290000 - 1 388342 L2035 Sep 2016 Twin (p) 2 3756801695685 · 2666669 - 1 200700 L1921 Dec 2011 Twin (p) 3 66982155216384 - 6698215528192 - 1 144605 A18 Apr 2024 Twin (p) 4 22271030616384 - 2227103068192 - 1 136770 A13 Mar 2024 Twin (p) 5 65516468355 · 2333333 - 1 100355 L923 Aug 2009 Twin (p) 6 201926367 · 2266668 - 1 80284 A25 May 2024 Twin (p) 7 160204065 · 2262148 - 1 78923 L5115 Jul 2021 Twin (p) 8 18936119858192 - 18936119854096 - 1 76000 A13 Mar 2024 Twin (p) 9 15891732708192 - 15891732704096 - 1 75376 A22 Feb 2024 Twin (p) 10 9960942348192 - 9960942344096 - 1 73715 A18 Jan 2024 Twin (p) 11 8957215318192 - 8957215314096 - 1 73337 A7 Jan 2024 Twin (p) 12 7955076968192 - 7955076964096 - 1 72915 A5 Jan 2024 Twin (p) 13 6915957608192 - 6915957604096 - 1 72417 A13 Jan 2024 Twin (p) 14 6470208268192 - 6470208264096 - 1 72180 A5 Jan 2024 Twin (p) 15 6298136548192 - 6298136544096 - 1 72084 A5 Jan 2024 Twin (p) 16 5049833348192 - 5049833344096 - 1 71298 A7 Jan 2024 Twin (p) 17 3143057258192 - 3143057254096 - 1 69611 A7 Dec 2023 Twin (p) 18 1845340868192 - 1845340864096 - 1 67716 A5 Dec 2023 Twin (p) 19 14279340881715 · 2222333 - 1 66943 L5843 Nov 2023 Twin (p) 20 12770275971 · 2222225 - 1 66907 L527 Jul 2017 Twin (p)

Related Pages

• Twin Primes from the World of Mathematics
• The Prime Glossary's: Twin primes

References

Forbes97

T. Forbes, "A large pair of twin primes," Math. Comp., 66 (1997) 451-455.  MR 97c:11111

Abstract: We describe an efficient integer squaring algorithm (involving the fast Fourier transform modulo F₈) that was used on a 486 computer to discover a large pair of twin primes.

[The twin primes 6797727 · 2¹⁵³²⁸± 1 are found on a 486 microcomputer]

IJ96

K. Indlekofer and A. Járai, "Largest known twin primes," Math. Comp., 65 (1996) 427-428.  MR 96d:11009

Abstract: The numbers 697053813 · 2¹⁶³⁵²± 1 are twin primes.

PSZ90

B. K. Parady, J. F. Smith and S. E. Zarantonello, "Largest known twin primes," Math. Comp., 55 (1990) 381-382.  MR 90j:11013