twin prime
Twin primes are pairs of primes which differ by two. (This name was coined by Stäckel in 1916.) The first twin primes are {3,5}, {5,7}, {11,13,} and {17,19}. It has been conjectured that there are infinitely many twin primes (see the twin prime conjecture for further information). Using sieve techniques, it has been proven that the sum of the reciprocals of the twin primes converge (see Brun's constant).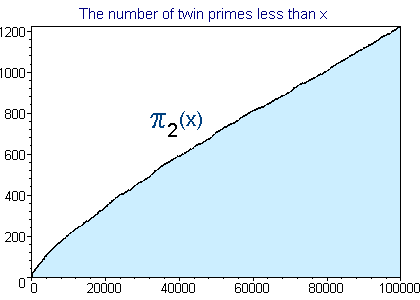
It is trivial to show that other than the first pair, all pairs of twin primes have the form {6n-1, 6n+1}. It takes more work to prove this variant of Wilson's theorem:
- Theorem: (Clement 1949)
- The integers n, n+2, form a pair of twin
primes if and only if
4[(n-1)!+1] = -n (mod n(n+2)).
See the other entries and pages linked below for more technical information.
See Also: TwinPrimeConstant, BrunsConstant, PrimeKtupleConjecture
Related pages (outside of this work)
- Twin primes (records and research)
References:
- Brent75
- R. P. Brent, "Irregularities in the distribution of primes and twin primes," Math. Comp., 29 (1975) 43--56. MR 51:5522
- Clement1949
- P. A. Clement, "Congruences for sets of primes," Amer. Math. Monthly, 56 (1949) 23--25. MR 10,353f
- IJ99
- K. Indlekofer and A. Járai, "Largest known twins and Sophie Germain primes," Math. Comp., 68:227 (1999) 1317--1324. MR 99k:11013 (Annotation available)
- Nicely95
- T. Nicely, "Enumeration to 1014 of the twin primes and Brun's constant," Virginia Journal of Science, 46:3 (1995) 195--204. MR 97e:11014 (Abstract available) [Available at http://www.trnicely.net/index.html]
- Suzuki2000
- M. Suzuki, "Alternative formulations of the twin prime problem," Amer. Math. Monthly, 107:1 (2000) 55--56. MR 2000m:11007
- Wrench61
- J. W. Wrench, "Evaluation of Artin's constant and the twin-prime constant," Math. Comp., 15 (1961) 396--398. MR 23:A1619
Printed from the PrimePages <t5k.org> © Reginald McLean.