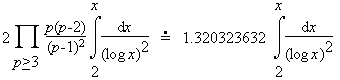twin prime constant

If we look at the number of twin primes less than x we see the usual irregularities associated with distributions of primes:

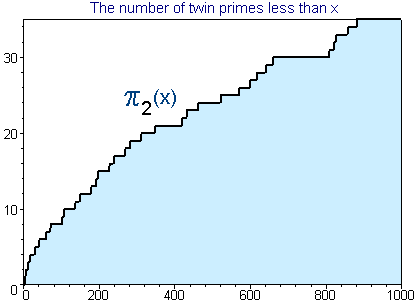
but back up and look again and the distribution looks much smoother:

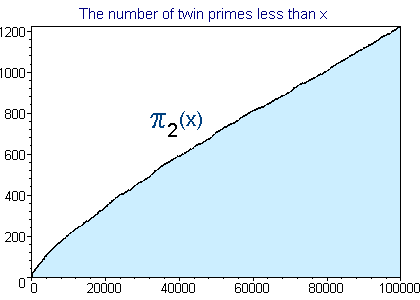
(Click here for a graph to 1,000,000).

Hardy and Littlewood conjectured that there are about

twin primes less than or equal to x where the infinite product is the twin prime constant. See the page "a simple heuristic" linked below for information on how this conjecture is formed.

This constant was estimated by Wrench and others to be approximately 0.6601618158...

See Also: TwinPrime, BrunsConstant, PrimeKtupleConjecture

Related pages (outside of this work)

• Twin primes (records and research)
• A simple heuristic