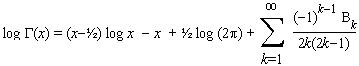Stirling's formula
Stirling found the following formula for approximating factorials:
and more precisely,
where 0 < ![]() < 1
(and e is the base of the natural logarithms).
In terms of the gamma function Stirling's formula is
< 1
(and e is the base of the natural logarithms).
In terms of the gamma function Stirling's formula is
and
where Bk is the kth Bernoulli number.
References:
- AS1974
- M. Abramowitz and I. Stegun editors, Handbook of mathematical functions--with formulas, graphs, and mathematical tables, Dover Pub., New York, NY, 1974. pp. xiv+1046, ISBN 0-486-61272-4. MR 94b:00012
Printed from the PrimePages <t5k.org> © Reginald McLean.