hypothesis H

In 1958 Schinzel and Sierpinski proposed the following generalization of Dickson's conjecture.

Conjecture (Hypothesis H)

Let k be a positive integer and let f₁(x), f₂(x), . . ., f_k(x) be irreducible polynomials with integral coefficients and positive leading coefficients.  Assume also that there is not a prime p which divides the product f₁(m)^.f₂(m)^. . . . ^. f_k(m) for every integer m.  Then there exists a positive integer n such that f₁(n), f₂(n), . . ., f_k(n) are all primes.

If there one such n making these polynomials all prime, then there are infinitely many such n.  So, for example, this conjecture implies there are infinitely many primes of the form n²+1.

Hypothesis H was quantified as follows by Bateman and Horn in 1962.  Let d_i be the degree of f_i.  For each prime p, let w(p) be the number of solutions to

f₁(n)^.f₂(n)^. . . . ^. f_k(n) ≡ 0 (mod p)

then the expected number of values of n less than N for which f₁(n), f₂(n), . . ., f_k(n) are simultaneously prime, is .

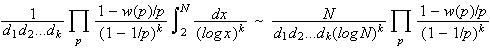References:

BH62

P. T. Bateman and R. A. Horn, "A heuristic asymptotic formula concerning the distribution of prime numbers," Math. Comp., 16 (1962) 363-367.  MR 26:6139

Ribenboim95 (chapter 6, section IV)

P. Ribenboim, The new book of prime number records, 3rd edition, Springer-Verlag, New York, NY, 1995.  pp. xxiv+541, ISBN 0-387-94457-5. MR 96k:11112 [An excellent resource for those with some college mathematics. Basically a Guinness Book of World Records for primes with much of the relevant mathematics. The extensive bibliography is seventy-five pages.]

SS58

A. Schinzel and W. Sierpinski, "Sur certaines hypotheses concernment les nombres premiers," Acta. Arith., 4 (1958) 185-208.  Erratum 5 (1958).