
1.The fundamental frequency of a stretched string is inversely proportional to the length of the string, keeping the tension and the mass per unit length of the string constant:

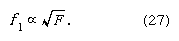
3.The fundamental frequency of a stretched string is inversely proportional to the mass per unit length of the string, keeping the length and the tension in the string constant:
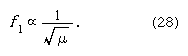
Mersenne's laws help explain the construction and operation of string instruments. The lower strings of a guitar or violin are made with a greater mass per unit length, and the higher strings made thinner and lighter. This means that the tension in all the strings can be made more nearly the same, resulting in a more uniform sound. In a grand piano, the tension in each string is over 100 pounds, creating a total force on the frame of between 40,000 and 60,000 pounds. A large variation in tension between the lower and the higher strings could lead to warping of the piano frame, so that, in order to apply even tension throughout, the higher strings are shorter and smaller in diameter while the bass strings are constructed of heavy wire wound with additional thin wire. This construction makes the wires stiff, causing the overtones to be higher in frequency than the ideal harmonics and leading to the slight inharmonicity that plays an important part in the characteristic piano tone.